X-ray crystallography

X-ray crystallography is a way to see the three-dimensional structure of a molecule. The electron cloud of an atom bends the X-rays slightly. This makes a "picture" of the molecule that can be seen on a screen. It can be used for both organic and inorganic molecules. The sample is not destroyed in the process.
Science writer Maggie Koerth (Maggie Koerth's page on regular Wikipedia) provides an analogy:
Imagine that you have captured Wonder Woman's invisible airplane. You can't see it. But you know it's there because when you throw a rubber ball at the space, the ball bounces back to you. If you could throw enough rubber balls, from all different sides, and measure their trajectory and speed as they bounced back, you could probably get a pretty good idea of the shape of the plane.[1]
X-ray crystallography was jointly invented by Sir William Bragg (1862–1942) and his son Sir Lawrence Bragg (1890–1971). They won the Nobel Prize in Physics for 1915. Lawrence Bragg is the youngest to be made a Nobel Laureate. He was the Director of the Cavendish Laboratory, Cambridge University, when the discovery of the structure of DNA was made by James D. Watson , Francis Crick , Maurice Wilkins, and Rosalind Franklin in February 1953.
The oldest method of X-ray crystallography is X-ray diffraction (XRD). X-rays are fired at a single crystal and the way they are scattered produces a pattern. These patterns are used to work out the arrangement of atoms inside the crystal.[2]
X-ray analysis of crystals[change | change source]
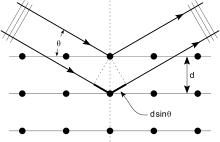
If atoms are arranged symmetrically with a separation d, these spherical waves will add up only where their path-length difference 2d sin θ equals a multiple of the wavelength λ. In that case, a reflection spot occurs in the diffraction pattern
Crystals are regular arrays of atoms, meaning that the atoms are repeated over and over in all three dimensions. X-rays are waves of electromagnetic radiation. When X-rays meet atoms, the electrons in the atoms cause the X-rays to scatter in all directions. Because the X-rays are emitted in all directions, an X-ray striking an electron produces secondary spherical waves emanating from the electron. The electron is known as the scatterer. A regular array of scatterers (here the repeating pattern of atoms in the crystal) produces a regular array of spherical waves. Although these waves cancel one another out in most directions, they add up in a few specific directions, determined by Bragg's law:
Here d is the spacing between diffracting planes, is the incident angle, n is any integer, and λ is the wavelength of the beam. These specific directions appear as spots on the diffraction pattern called reflections. Thus, X-ray diffraction results from an electromagnetic wave (the X-ray) hitting a regular array of scatterers (the repeating arrangement of atoms within the crystal).
Related pages[change | change source]
References[change | change source]
- ↑ Koerth, Maggie (November 8, 2012). "The Turn of the Screw: James Watson on The Double Helix and his changing view of Rosalind Franklin". Boing Boing.
- ↑ "Introduction to X-ray Diffraction (XRD)". panalytical.com. 2012. Archived from the original on 4 August 2012. Retrieved 6 November 2012.


